Let α>0 be a (fixed) real number.

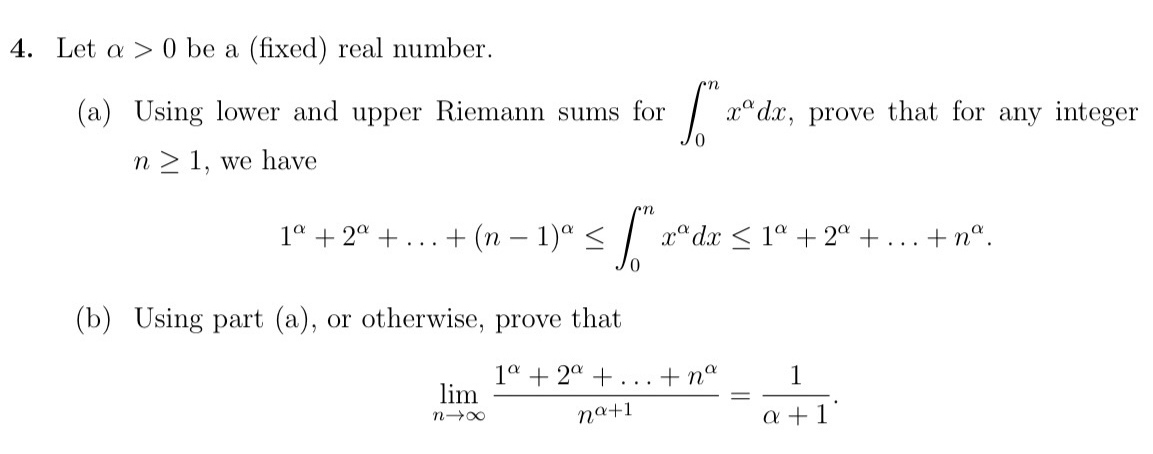
Let $\alpha>0$ be a (fixed) real number.
(a) Using lower and upper Riemann sums for $\int_0^n x^\alpha d x$, prove that for any integer $n \geq 1$, we have

$$ 1^\alpha+2^\alpha+\ldots+(n-1)^\alpha \leq \int_0^n x^\alpha d x \leq 1^\alpha+2^\alpha+\ldots+n^\alpha $$

(b) Using part (a), or otherwise, prove that

$$ \lim _{n \rightarrow \infty} \frac{1^\alpha+2^\alpha+\ldots+n^\alpha}{n^{\alpha+1}}=\frac{1}{\alpha+1} $$