Let I=∫_0^1 arctan (x)/x d x

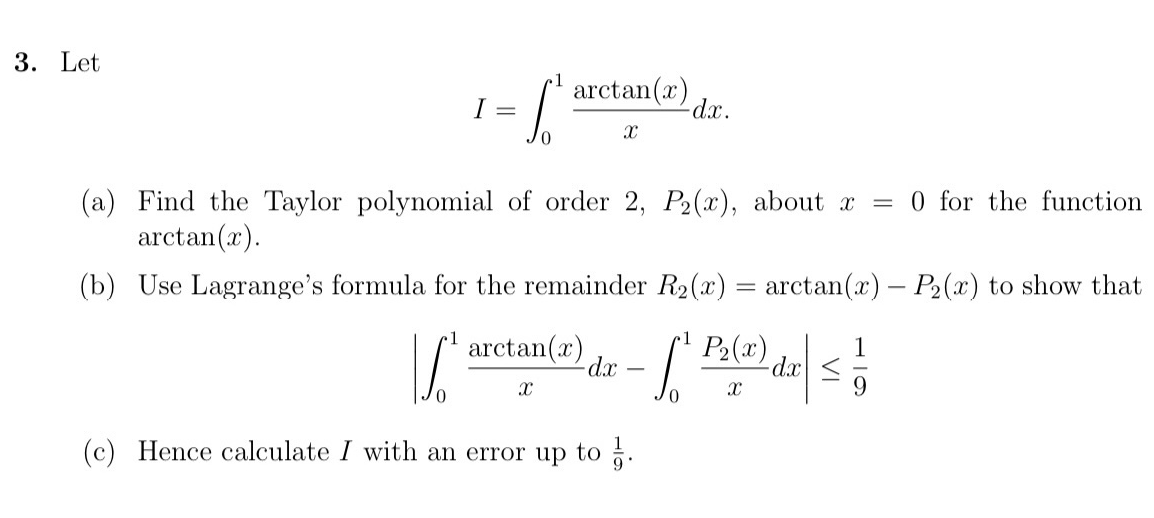
Let

$$ I=\int_0^1 \frac{\arctan (x)}{x} d x $$

(a) Find the Taylor polynomial of order $2, P_2(x)$, about $x=0$ for the function $\arctan (x)$
(b) Use Lagrange's formula for the remainder $R_2(x)=\arctan (x)-P_2(x)$ to show that

$$ \left|\int_0^1 \frac{\arctan (x)}{x} d x-\int_0^1 \frac{P_2(x)}{x} d x\right| \leq \frac{1}{9} $$

(c) Hence calculate $I$ with an error up to $\frac{1}{9}$.